BREMIC

Ocean Model Description

The ocean model is based on the Hamburg large-scale geostrophic ocean model LSG (Maier-Reimer et al. 1993). The model integrates the momentum equations, including all terms except the nonlinear advection of momentum, by an implicit time integration method which allows a time step of 1 month. The free surface is treated prognostically. The model has 11 vertical levels and a horizontal resolution of 3.5 $^{\circ}$ on a semi-staggered grid type "E" (cf. Mesinger & Arakawa 1976). A new tracer advection scheme for temperature and salinity has been implemented. It is an adaption of the scheme by Farrow & Stevens (1995) using a predictor-corrector method, with a centered difference scheme for the predictor and a third-order QUICK scheme (Leonard 1979; Schäfer-Neth & Paul 2001) for the corrector stage. Explicit integration of both stages results in a time step constraint that requires the use of subcycles. Here we employ 8 subcycles, i.e. a time step of 3.75 days for tracer advection. The QUICK scheme is less diffusive than the standard LSG upstream scheme and less dispersive than the common centered difference scheme.

The ocean model is driven by monthly fields of wind stress, surface air temperature and freshwater flux provided by the atmospheric GCM ECHAM3/T42 (Roeckner et al. 1992). In order to close the hydrological cycle, a runoff scheme transports freshwater from the continents to the ocean. For the surface heat flux Q we use a boundary condition of the form

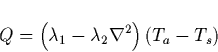$\begin{displaymath} Q = \left(\lambda_1-\lambda_2\nabla^2\right) \left(T_a-T_s\right) \end{displaymath}$ (1)

as suggested by Willebrand (1993). Here, T_a is the prescribed air temperature, and T_s denotes the ocean surface temperature. Unlike conventional temperature restoring, the thermal boundary condition (1) allows for scale selective damping of surface temperature anomalies. For the parameters $\lambda_1$ and $\lambda_2$ we choose 15 W m^-2 K^-1 and $2\cdot 10^{12}$ W K^-1, respectively. This choice enables the simulation of observed sea surface temperatures as well as the maintenance of large-scale temperature anomalies in the North Atlantic during perturbation experiments, which is demonstrated in the following.

Influence of the surface heat flux parameters $\lambda_1$ and $\lambda_2$ on sea surface temperatures and THC stability

The surface heat flux formulation (1) allows for scale selective damping of sea surface temperature anomalies, and is therefore superior to the conventional restoring approach. Applying $\lambda_1 = 15$ W m^-2 K^-1 and $\lambda_2 = 2\cdot 10^{12}$ W K^-1 in our model set-up, the time scale for surface restoring can vary from one month for small-scale temperature anomalies to almost half a year for large-scale anomalies. It is important that these parameters allow the simulation of sea surface temperatures which are close to observations (a: Model, b: Levitus observational data):

It is well known that surface heat flux parameterizations have a substantial influence on the stability properties of the THC. In particular, a strong damping of surface temperature anomalies can considerably decrease the stability of the "on" mode (e.g., Mikolajewicz & Maier-Reimer 1994; Rahmstorf & Willebrand 1995; Lohmann et al. 1996; Prange et al. 1997). Therefore, we carried out sensitivity experiments to demonstrate the effects of $\lambda_1$ and $\lambda_2$ on THC stability.

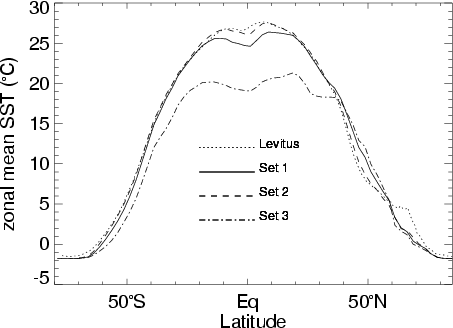
The figure above shows zonal mean sea surface temperatures using three different sets of heat flux parameters. Standard values (see above) are used in set 1. Set 2 defines a conventional restoring approach, where $\lambda_2$ is set to zero, and $\lambda_1 = 75$ W m^-2 K^-1. With a thickness of 50 m for the topmost boxes of the model grid, this corresponds to a restoring time scale of approximately one month. Set 3 consists of values suggested by Rahmstorf & Willebrand (1995), i.e. $\lambda_1 = 3$ W m^-2 K^-1 and $\lambda_2 = 8\cdot 10^{12}$ W K^-1. These authors have shown that the thermal boundary condition (1) can be derived from an atmospheric energy balance model with diffusive lateral heat transport. In their derivation, the restoring temperature is strictly defined as the surface temperature that would be reached in the absence of oceanic heat transports. Using actual air temperatures, as we do in our set-up, the heat flux parameters of Rahmstorf & Willebrand (1995) are not appropriate, resulting in strongly reduced surface temperatures in low latitudes.

The model's capability to maintain sea surface temperature anomalies is demonstrated in the figures below for the different sets of heat flux parameters. Applying set 1 or set 3, we find considerable surface temperature differences between "off" mode and "on" mode in the North Atlantic. The strong restoring of set 2, however, suppresses the development of temperature anomalies. North Atlantic cooling tends to stabilize the "on" mode by increasing the density of North Atlantic water masses. This is clearly expressed in the stability diagram.

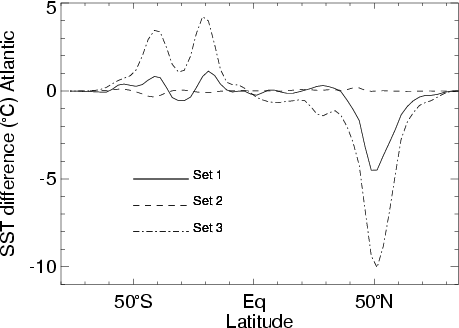
We conclude that our standard set of parameters ( $\lambda_1 = 15$ W m^-2 K^-1, $\lambda_2 = 2\cdot 10^{12}$ W K^-1) is a suitable choice, allowing the simulation of observed sea surface temperatures and the maintenance of large-scale temperature anomalies in perturbation experiments.